First work3 code version and report initialization

Work 3/report/Work3_report.tex

@@ -0,0 +1,93 @@
+%
+% Optimization Techniques Work 3 report
+%
+% authors:
+%   Χρήστος Χουτουρίδης ΑΕΜ 8997
+%   cchoutou@ece.auth.gr
+
+
+\documentclass[a4paper, 11pt]{AUTHReport}
+
+% Document configuration
+\AuthorName{Χρήστος Χουτουρίδης}
+\AuthorAEM{8997}
+\AuthorMail{cchoutou@ece.auth.gr}
+
+%\CoAuthorName{CoAuthor Name}
+%\CoAuthorAEM{AEM}
+%\CoAuthorMail{CoAuthor Mail}
+
+% \WorkGroup{Ομάδα Χ}
+
+\DocTitle{3η Εργαστηριακή Άσκηση}
+\DocSubTitle{Μέθοδος Μέγιστης Καθόδου με Προβολή}
+
+\Department{Τμήμα ΗΜΜΥ. Τομέας Ηλεκτρονικής}
+\ClassName{Τεχνικές Βελτιστοποίησης}
+
+\InstructorName{Γ. Ροβιθάκης}
+\InstructorMail{rovithak@auth.gr}
+
+\CoInstructorName{Θ. Αφορόζη}
+\CoInstructorMail{taforozi@ece.auth.gr}
+
+\CurrentDate{\today}
+
+
+\usepackage{enumitem}
+\usepackage{tabularx}
+\usepackage{array}
+\usepackage{amssymb}
+\usepackage{amsfonts}
+\usepackage{amsmath}
+\usepackage{commath}
+
+\usepackage{float}
+
+
+\begin{document}
+
+\InsertTitle
+
+%\tableofcontents
+
+\sloppy
+
+\section{Εισαγωγή}
+Η παρούσα εργασία αφορά το πρόβλημα της ελαχιστοποίησης μιας δοσμένης συνάρτησης πολλών μεταβλητών $f: \mathbb{R}^n \rightarrow \mathbb{R}$ χωρίς περιορισμούς.
+Για το σκοπό αυτό κάνουμε χρήση τριών μεθόδων.
+Της μεθόδου μέγιστης καθόδου (Steepest Descent), της μεθόδου Newton, και της Levenberg-Marquardt.
+Ακόμα για κάθε μία από αυτές θα υλοποιήσουμε τρεις διαφορετικές τεχνικές υπολογισμού βήματος.
+
+\section{Παραδοτέα}
+Τα παραδοτέα της εργασίας αποτελούνται από:
+\begin{itemize}
+    \item Την παρούσα αναφορά.
+    \item Τον κατάλογο \textbf{scripts/}, που περιέχει τον κώδικα της MATLAB.
+    \item Το \href{https://git.hoo2.net/hoo2/OptimizationTechniques/src/branch/master/Work%203}{σύνδεσμο} με το αποθετήριο που περιέχει όλο το project με τον κώδικα της MATLAB, της αναφοράς και τα παραδοτέα.
+\end{itemize}
+
+\section{Προγραμματιστική προσέγγιση}
+Για τον προγραμματισμό και εκτέλεση των μεθόδων της παρούσας εργασίας έγινε χρήση της MATLAB.
+Στον κατάλογο \textbf{scripts}, περιέχονται όλες οι μέθοδοι και οι τεχνικές υπολογισμού βημάτων με τη μορφή συναρτήσεων καθώς και scripts που τις καλούν.
+Για κάθε μία μέθοδο (ένα θέμα της εργασίας), υπάρχει το αντίστοιχο script που περιέχει τους υπολογισμούς, τις κλήσεις των μεθόδων και τη δημιουργία των διαγραμμάτων.
+Για το πρώτο θέμα το αρχείο Script\_1\_Plots.m για το δεύτερο το Script\_2\_Steepest\_descent.m και ούτω καθεξής.
+Στην παρούσα εργασία η υλοποίηση του κώδικα ακολουθεί την τεχνική της προηγούμενης εργασίας και “ομαδοποιεί” αρκετές λειτουργίες.
+Πιο συγκεκριμένα.
+
+\subsection{Symbolic expression functions}
+Μία ακόμη προγραμματιστική τεχνική που ακολουθήθηκε είναι η χρήση \textbf{symbolic expression} για την αναπαράσταση των διαφορετικών αντικειμενικών συναρτήσεων.
+Ο λόγος που επιλέχθηκε είναι η \textbf{δυνατότητα εξαγωγής ενός symbolic expression που αναπαριστά την κλίση $\nabla f$ και τον Εσσιανό $\nabla^2f$  μιας συνάρτησης} από την MATLAB, κάνοντας χρήση των εντολών \textit{gradient()} και \textit{hessian()}.
+Αν αντίθετα χρησιμοποιούσαμε απλές συναρτήσεις, πολυώνυμα ή lambdas για την αναπαράσταση των αντικειμενικών συναρτήσεων, τότε για τον υπολογισμό της κλίσης και του Εσσιανού θα έπρεπε:
+\begin{itemize}
+    \item Είτε να υπολογίζαμε αριθμητικά τις παραγώγους gradient και hessian μέσα στις μεθόδους, κάτι που θα εισήγαγε \textit{\textbf{αχρείαστο αριθμητικό σφάλμα}}.
+    \item Είτε να κάναμε χρήση δύο επιπλέων συναρτήσεων (ή πολυωνύμων) για την αναπαράσταση τους, κάτι που ουσιαστικά θα δημιουργούσε \textit{\textbf{πλεονασμό πληροφορίας εισόδου}} και άρα μεγαλύτερη πιθανότητα να κάνουμε λάθος.
+\end{itemize}
+Η αναπαράσταση όμως με χρήση symbolic expression είναι πιο “βαριά” όταν χρειάζεται να υπολογίσουμε την τιμή μιας συνάρτησης σε κάποιο σημείο (subs(expr, number)).
+Αυτό είναι κάτι που χρειάζεται εκτενώς στον κώδικά μας.
+Για το λόγο αυτό, ενώ η συνάρτηση δίνεται ως symbolic expression, μέσω αυτής υπολογίζονται αυτόματα η κλίση, ο Εσσιανός αλλά και οι “κανονικές” συναρτήσεις MATLAB που τις υλοποιούν.
+Έτσι έχουμε την ακριβή αναπαράσταση της κλίσης και του Εσσιανού ως συναρτήσεις χωρίς να πληρώνουμε το κόστος της subs().
+
+
+
+\end{document}

Work 3/scripts/BelongsTo.m

@@ -0,0 +1,19 @@
+function [flag] = BelongsTo(x, SetLimmits)
+%Checks if the x vector belongs to Set
+%
+% x:          A vector to project
+% SetLimmits: The set to project. Each line/dimension off the set has to contain the limits
+%             of the set to that particular dimension
+% flag:       True if belongs
+%
+    flag = true;    % Have faith
+
+    for i = 1:size(SetLimmits, 2)
+        if x(i) < SetLimmits(i,1)
+            flag = false;
+        elseif x(i) > SetLimmits(i,2)
+            flag = false;
+        end
+    end
+end
+

Work 3/scripts/GivenEnv.asv

@@ -0,0 +1,33 @@
+% Given environment
+
+clear;
+% Setup the function under test
+syms x [2 1] real;
+fexpr = (1/3)*x(1)^2 +3*x(2)^2;
+title_fun = "$f(x) = frac{1}{3}{x_1}^2 + 3{x_2}^2$";
+
+% Calculate the gradient and Hessian
+grad_fexpr = gradient(fexpr, x); % Gradient of f
+hessian_fexpr = hessian(fexpr, x);   % Hessian of f
+
+% Convert symbolic expressions to MATLAB functions
+fun         = matlabFunction(fexpr, 'Vars', {x});        % Function
+grad_fun    = matlabFunction(grad_fexpr, 'Vars', {x}); % Gradient
+hessian_fun = matlabFunction(hessian_fexpr, 'Vars', {x});  % Hessian
+
+% Minimum reference
+%Freference = @(x) x(1).^5 .* exp(-x(1).^2 - x(2).^2);
+%[Xmin, Fmin] = fminsearch(Freference, [-1, -1]);
+
+% Amijo globals
+global amijo_beta; % Step reduction factor in [0.1, 0.5] (typical range: [0.1, 0.8])
+global amijo_sigma; % Sufficient decrease constant in [1e-5, 0.1] (typical range: [0.01, 0.3])
+
+%fixed step size globals
+global  gamma_fixed_step
+
+global image_width, 
+global image_height;
+
+image_width = 960;
+image_height = 640;

Work 3/scripts/GivenEnv.m

@@ -0,0 +1,36 @@
+% Given environment
+
+clear;
+% Setup the function under test
+syms x [2 1] real;
+fexpr = (1/3)*x(1)^2 +3*x(2)^2;
+title_fun = "$f(x) = \frac{1}{3}{x_1}^2 + 3{x_2}^2$";
+
+XSetLimmits = [-10, 5 ; -8, 12];
+
+% Calculate the gradient and Hessian
+grad_fexpr = gradient(fexpr, x); % Gradient of f
+hessian_fexpr = hessian(fexpr, x);   % Hessian of f
+
+% Convert symbolic expressions to MATLAB functions
+fun         = matlabFunction(fexpr, 'Vars', {x});         % Function
+grad_fun    = matlabFunction(grad_fexpr, 'Vars', {x});    % Gradient
+hessian_fun = matlabFunction(hessian_fexpr, 'Vars', {x}); % Hessian
+
+% Minimum reference
+[Xmin, Fmin] = fminsearch(fun, [-1, -1]');
+Xmin = round(Xmin, 3);
+Fmin = round(Fmin, 3);
+
+% Amijo globals
+global amijo_beta; % Step reduction factor in [0.1, 0.5] (typical range: [0.1, 0.8])
+global amijo_sigma; % Sufficient decrease constant in [1e-5, 0.1] (typical range: [0.01, 0.3])
+
+%fixed step size globals
+global  gamma_fixed_step
+
+global image_width, 
+global image_height;
+
+image_width = 960;
+image_height = 640;

Work 3/scripts/ProjectionPoint.m

@@ -0,0 +1,18 @@
+function [x_p] = ProjectionPoint(x, SetLimmits)
+%Project the x vector to Set, returns a point of Set close(st) to x
+%
+% x:          A vector to project
+% SetLimmits: The set to project. Each line/dimension off the set has to contain the limits
+%             of the set to that particular dimension
+%x_p:         The projected point
+%
+    x_p = x;
+    for i = 1:size(SetLimmits, 2)
+        if x(i) < SetLimmits(i,1)
+            x_p(i) = SetLimmits(i,1);
+        elseif x(i) > SetLimmits(i,2)
+            x_p(i) = SetLimmits(i,2);
+        end
+    end
+end
+

Work 3/scripts/Script_0_Plots.m

@@ -0,0 +1,14 @@
+% Define environment (functions, gradients etc...)
+GivenEnv
+
+%
+% We plot the function in the domain of x,y in [-3, 3].
+% We also plot the contour in order to get a sense of the min and maximum
+% points in the x-y plane
+%
+
+% 3d plot the function
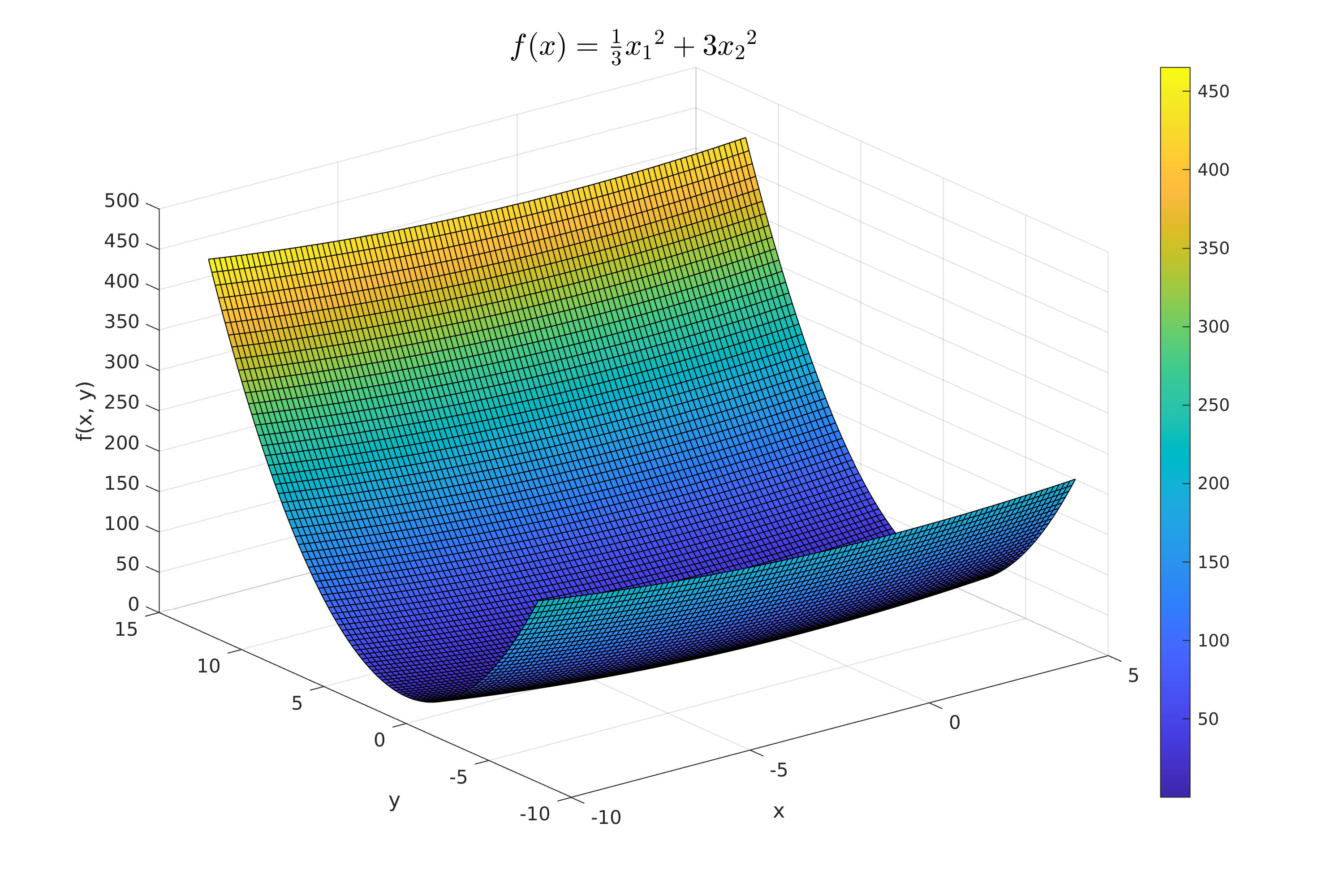
+plot3dFun(fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, title_fun, "figures/Plot_Function.png");
+
+% Plot isobaric lines
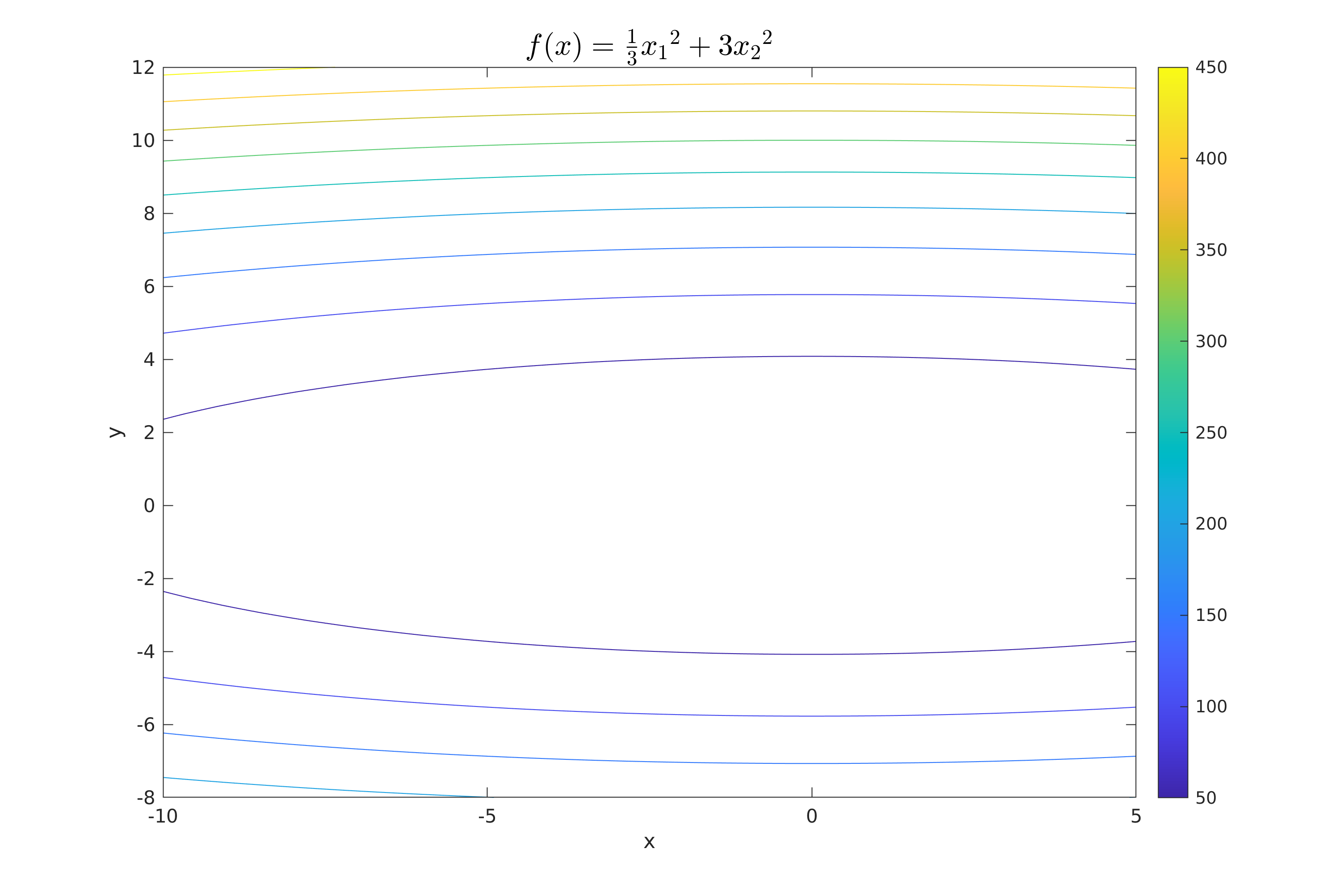
+plotContour(fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, title_fun, "figures/Plot_Contour.png");

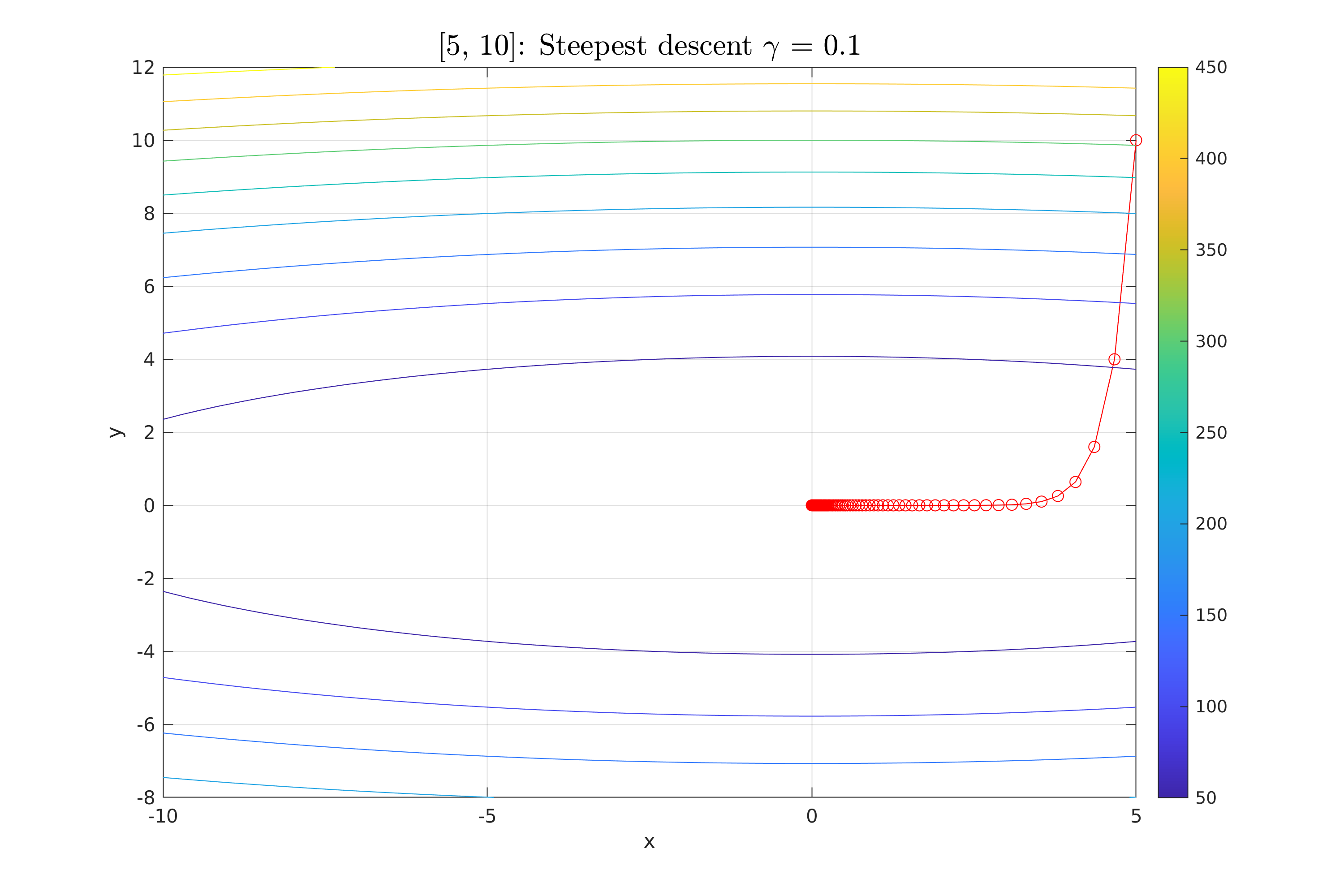
Work 3/scripts/Script_1_SteepDesc.m

@@ -0,0 +1,29 @@
+% Define environment (functions, gradients etc...)
+GivenEnv
+
+% Define parameters
+max_iter = 1000; % Maximum iterations
+tol = 1e-4; % Tolerance
+
+% Point x0 = (1, 1)
+% =========================================================================
+point = 1;
+x0 = [5, -5]';
+point_str = "[" + x0(1) + ", " + x0(2) + "]";
+f = fun(x0);
+gf = grad_fun(x0);
+hf = hessian_fun(x0);
+fprintf('Initial point (%d, %d), f = %f, grad = [%f;%f], hessian = [%f %f ; %f %f]=> Method applicable\n', x0, f, gf, hf);
+
+for g=[0.1, 0.3, 0.5, 3, 5]
+    gamma_fixed_step = g;
+
+    [x_fixed, f_fixed, kk] = method_SteepDesc(fun, grad_fun, x0, tol, max_iter, 'fixed');
+    fprintf('Fixed step g=%f:     Initial point (%f, %f), steps:%d, Final (x1,x2)=(%f, %f), f(x1,x2)=%f\n', g, x0, kk, x_fixed(:, end), f_fixed(end));
+    if g <= 1
+        plotPointsOverContour(x_fixed, fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, point_str + ": Steepest descent $\gamma$ = " + gamma_fixed_step, "");
+    else
+        plotPointsOverContour(x_fixed, fun, [-500, 500], [-500, 500], 100, point_str + ": Steepest descent $\gamma$ = " + gamma_fixed_step, "");
+    end
+end
+

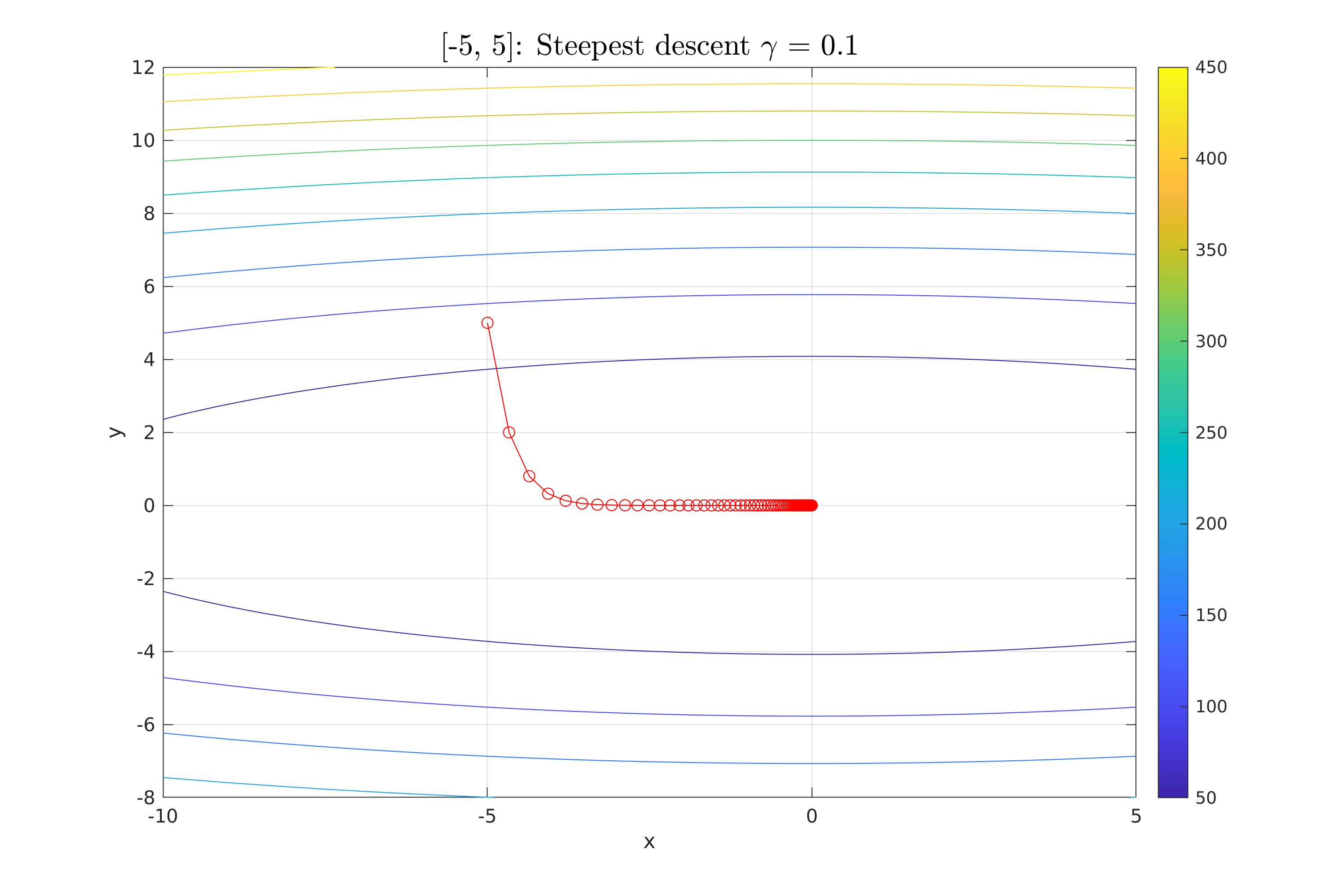
Work 3/scripts/Script_2_SteepDesc_Proj.m

@@ -0,0 +1,32 @@
+% Define environment (functions, gradients etc...)
+GivenEnv
+
+% Settings
+max_iter = 1000; % Maximum iterations
+
+% Define parameters
+% =========================================================================
+x0 = [5, -5]';
+gamma = 0.5;
+sk_step = 5;
+tol = 0.01; % Tolerance
+
+
+
+point_str = "[" + x0(1) + ", " + x0(2) + "]";
+f = fun(x0);
+gf = grad_fun(x0);
+hf = hessian_fun(x0);
+fprintf('Initial point (%d, %d), f = %f, grad = [%f;%f], hessian = [%f %f ; %f %f]=> Method applicable\n', x0, f, gf, hf);
+
+
+gamma_fixed_step = gamma;
+[x_fixed, f_fixed, kk] = method_SteepDesc_Proj(fun, grad_fun, x0, sk_step, XSetLimmits, tol, max_iter, 'fixed');
+fprintf('Fixed step g=%f:     Initial point (%f, %f), steps:%d, Final (x1,x2)=(%f, %f), f(x1,x2)=%f\n', gamma_fixed_step, x0, kk, x_fixed(:, end), f_fixed(end));
+plotPointsOverContour(x_fixed, fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, point_str + ": Steepest descent $\gamma$ = " + gamma_fixed_step, "");
+
+%[x_minimized, f_minimized, kk] = method_SteepDesc_Proj(fun, grad_fun, x0, sk_step, XSetLimmits, tol, max_iter, 'minimized');
+%fprintf('Minimized f:         Initial point (%f, %f), steps:%d, Final (x1,x2)=(%f, %f), f(x1,x2)=%f\n', x0, kk, x_fixed(:, end), f_fixed(end));
+%plotPointsOverContour(x_fixed, fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, point_str + ": Steepest descent minimized", "");
+
+

Work 3/scripts/Script_3_SteepDesc_Proj.m

@@ -0,0 +1,27 @@
+% Define environment (functions, gradients etc...)
+GivenEnv
+
+% Settings
+max_iter = 1000; % Maximum iterations
+
+% Define parameters
+% =========================================================================
+x0 = [-5, 10]';
+gamma = 0.1;
+sk_step = 55;
+tol = 0.01; % Tolerance
+
+
+
+point_str = "[" + x0(1) + ", " + x0(2) + "]";
+f = fun(x0);
+gf = grad_fun(x0);
+hf = hessian_fun(x0);
+fprintf('Initial point (%d, %d), f = %f, grad = [%f;%f], hessian = [%f %f ; %f %f]=> Method applicable\n', x0, f, gf, hf);
+
+
+gamma_fixed_step = gamma;
+[x_fixed, f_fixed, kk] = method_SteepDesc_Proj(fun, grad_fun, x0, sk_step, XSetLimmits, tol, max_iter, 'fixed');
+fprintf('Fixed step g=%f:     Initial point (%f, %f), steps:%d, Final (x1,x2)=(%f, %f), f(x1,x2)=%f\n', gamma_fixed_step, x0, kk, x_fixed(:, end), f_fixed(end));
+plotPointsOverContour(x_fixed, fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, point_str + ": Steepest descent $\gamma$ = " + gamma_fixed_step, "");
+

Work 3/scripts/Script_4_SteepDesc_Proj.m

@@ -0,0 +1,27 @@
+% Define environment (functions, gradients etc...)
+GivenEnv
+
+% Settings
+max_iter = 1000; % Maximum iterations
+
+% Define parameters
+% =========================================================================
+x0 = [8, -10]';
+gamma = 0.2;
+sk_step = 0.1;
+tol = 0.01; % Tolerance
+
+
+
+point_str = "[" + x0(1) + ", " + x0(2) + "]";
+f = fun(x0);
+gf = grad_fun(x0);
+hf = hessian_fun(x0);
+fprintf('Initial point (%d, %d), f = %f, grad = [%f;%f], hessian = [%f %f ; %f %f]=> Method applicable\n', x0, f, gf, hf);
+
+
+gamma_fixed_step = gamma;
+[x_fixed, f_fixed, kk] = method_SteepDesc_Proj(fun, grad_fun, x0, sk_step, XSetLimmits, tol, max_iter, 'fixed');
+fprintf('Fixed step g=%f:     Initial point (%f, %f), steps:%d, Final (x1,x2)=(%f, %f), f(x1,x2)=%f\n', gamma_fixed_step, x0, kk, x_fixed(:, end), f_fixed(end));
+plotPointsOverContour(x_fixed, fun, XSetLimmits(1, :), XSetLimmits(2, :), 100, point_str + ": Steepest descent $\gamma$ = " + gamma_fixed_step, "");
+

Work 3/scripts/fmin_bisection.m

@@ -0,0 +1,49 @@
+function [a, b, k, n] = fmin_bisection(fun, alpha, beta, epsilon, lambda)
+% Bisection method for finding the local minimum of a function.
+%
+% fun:      The objective function
+% alpha:    (number) The starting point of the interval in which we seek
+%           for minimum
+% beta:     (number) The ending point of the interval in which we seek
+%           for minimum
+% epsilon:  (number) The epsilon value (distance from midpoint)
+% lambda:   (number) The lambda value (accuracy)
+%
+% return:
+%  a:       (vector) Starting points of the interval for each iteration
+%  b:       (vector) Ending points of the interval for each iteration
+%  k:       (number) The number of iterations
+%  n:       (number) The calls of objective function fun_expr
+%
+
+
+
+% Error checking
+if alpha > beta || 2*epsilon >= lambda || lambda <= 0
+    error ('Input criteria not met')
+end
+
+% Init
+a = alpha;
+b = beta;
+n = 0;
+
+k=1;
+while b(k) - a(k) > lambda
+    % bisect [a,b]
+    mid = (a(k) + b(k)) / 2;
+    x_1 = mid - epsilon;
+    x_2 = mid + epsilon;
+    
+    % set new search interval
+    k = k + 1;
+    if fun(x_1) < fun(x_2)
+        a(k) = a(k-1);
+        b(k) = x_2;
+    else
+        a(k) = x_1;
+        b(k) = b(k-1);
+    end
+end
+
+end

Work 3/scripts/gamma_armijo.m

@@ -0,0 +1,32 @@
+function [gamma] = gamma_armijo(f, grad_f, dk, xk)
+    % Calculates the best step based on amijo method
+    %
+    % f(xk​+ γk*dk) ≤ f(xk) + σ * γk * dk^T * ∇f(xk)
+    % γk = β*γk_0
+    %
+    % f:        Objective function
+    % grad_fun: Gradient function of f
+    % dk:       Current value of selected direction -∇f or -inv{H}*∇f or -inv{H + lI}*∇f
+    % xk:       Current point (x,y)
+    
+    
+    % beta:     beta  factor in [0.1, 0.5]
+    % signam:   sigma factor in (0, 0.1]
+    global amijo_beta
+    global amijo_sigma
+
+    gf = grad_f(xk);
+    gamma = 1; % Start with a step size of 1
+
+    % Perform Armijo line search
+    while f(xk + gamma * dk) > f(xk) + amijo_sigma * gamma * dk * gf
+    %while f(xk(1) + gamma * dk(1), xk(2) + gamma * dk(2)) > ...
+    %      f(xk(1), xk(2)) + amijo_sigma * gamma * norm(dk)^2
+        gamma = amijo_beta * gamma; % Reduce step size
+        if gamma < 1e-12 % Safeguard to prevent infinite reduction
+            warning('Armijo step size became too small.');
+            break;
+        end
+    end
+
+end

Work 3/scripts/gamma_fixed.m

@@ -0,0 +1,12 @@
+function [gamma] = gamma_fixed(~, ~, ~, ~)
+    % Return a fixed step
+    %
+    % This is for completion and code symmetry. 
+    %
+    
+    global gamma_fixed_step
+    
+    % Perform line search
+    gamma = gamma_fixed_step;
+
+end

Work 3/scripts/gamma_minimized.m

@@ -0,0 +1,24 @@
+function [gamma] = gamma_minimized(f, ~, dk, xk)
+    % Calculates the step based on minimizing f(xk​− γk*dk)
+    %
+    %
+    % f:    Objective function
+    % ~:    Gradient function of f - Not used
+    % dk:       Current value of selected direction -∇f or -inv{H}*∇f or -inv{H + lI}*∇f
+    % xk:   Current point (x,y)
+
+    % Define the line search function fmin(g) = f(xk - g * dk)
+    fmin = @(g) f(xk) + g * dk;
+
+    % find g that minimizes fmin
+    e = 0.0001;
+    l = 0.001;
+    [a,b,k,~] = fmin_bisection(fmin, 0.0001, 1, e, l);   % g in (0, 1]
+    gamma = 0.5*(a(k) + b(k));
+
+    % Define the line search function fmin(g) = f(xk - g * dk)
+    %fmin = @(g) f(xk(1) - gamma * dk(1), xk(2) - gamma * dk(2));
+
+    % find g that minimizes fmin
+    %gamma = fminbnd(g, 0, 1);
+end

Work 3/scripts/method_SteepDesc.m

@@ -0,0 +1,39 @@
+function [x_vals, f_vals, k] = method_SteepDesc(f, grad_f, xk, tol, max_iter, mode)
+    % f:        Objective function
+    % grad_f:   Gradient of the function
+    % xk:       Initial point [x0; y0]
+    % tol:      Tolerance for stopping criterion
+    % max_iter: Maximum number of iterations
+    
+    % x_vals: Vector with the (x,y) values until minimum
+    % f_vals: Vector with f(x,y) values until minimum
+    % k:      Number of iterations
+    
+    if strcmp(mode, 'armijo') == 1
+        gamma_f = @(f, grad_f, dk, xk) gamma_armijo(f, grad_f, dk, xk);
+    elseif strcmp(mode, 'minimized') == 1
+        gamma_f = @(f, grad_f, dk, xk) gamma_minimized(f, grad_f, dk, xk);
+    else % mode == 'fixed'
+        gamma_f = @(f, grad_f, dk, xk) gamma_fixed(f, grad_f, dk, xk);
+    end
+    
+    % Storage for iterations, begin with the first point
+    x_vals = xk;
+    f_vals = f(xk);
+    for k = 1:max_iter
+        % Check for convergence
+        if norm(grad_f(xk)) < tol
+            break;
+        end
+        dk = - grad_f(xk);          % Steepset descent direction
+
+        gk = gamma_f(f, grad_f, dk, xk);   % Calculate gamma
+        
+        x_next = xk + gk * dk;      % Update step
+        f_next = f(x_next);
+        
+        xk = x_next;                % Update point
+        x_vals = [x_vals, x_next];  % Store values
+        f_vals = [f_vals, f_next];  % Store function values
+    end
+end

Work 3/scripts/method_SteepDesc_Proj.m

@@ -0,0 +1,50 @@
+function [x_vals, f_vals, k] = method_SteepDesc_Proj(f, grad_f, xk, sk, limmits, tol, max_iter, mode)
+    % f:        Objective function
+    % grad_f:   Gradient of the function
+    % xk:       Initial point [x0; y0]
+    % sk:       Step size (fixed positive scalar)
+    % limits:   Bounds of the feasible set for each dimension
+    % tol:      Tolerance for stopping criterion
+    % max_iter: Maximum number of iterations
+    
+    % x_vals: Vector with the (x,y) values until minimum
+    % f_vals: Vector with f(x,y) values until minimum
+    % k:      Number of iterations
+    
+    if strcmp(mode, 'armijo') == 1
+        gamma_f = @(f, grad_f, dk, xk) gamma_armijo(f, grad_f, dk, xk);
+    elseif strcmp(mode, 'minimized') == 1
+        gamma_f = @(f, grad_f, dk, xk) gamma_minimized(f, grad_f, dk, xk);
+    else % mode == 'fixed'
+        gamma_f = @(f, grad_f, dk, xk) gamma_fixed(f, grad_f, dk, xk);
+    end
+    
+    % Project the first point if needed
+    xk = ProjectionPoint(xk, limmits);
+
+    % Storage for iterations, begin with the first point
+    x_vals = xk;
+    f_vals = f(xk);
+    for k = 1:max_iter
+        % Check for convergence
+        if norm(grad_f(xk)) < tol
+            break;
+        end
+        dk = - grad_f(xk);          % Steepset descent direction
+
+        % First calculate xk-bar and project it if nessesary
+        xkbar = xk + sk * dk;
+        xkbar = ProjectionPoint(xkbar, limmits);
+
+        dk = (xkbar - xk);          % Steepest descent projection direction
+        
+        gk = gamma_f(f, grad_f, dk, xk);   % Calculate gamma
+        
+        x_next = xk + gk * dk;      % Update step
+        f_next = f(x_next);
+        
+        xk = x_next;                % Update point
+        x_vals = [x_vals, x_next];  % Store values
+        f_vals = [f_vals, f_next];  % Store function values
+    end
+end

Work 3/scripts/plot3dFun.m

@@ -0,0 +1,42 @@
+function plot3dFun(fun, x_lim, y_lim, size, plot_title, filename)
+    % 3D plots a function
+    % fun:          The function to plot
+    % x_lim:        The range for x axis. ex: [-2, 2]
+    % y_lim:        The range for y axis. ex: [0, 2]
+    % size:         The number of points for each axis
+    % plot_title:   The latex title for the plot
+    %
+
+    global image_width, 
+    global image_height;
+
+    % Generate a grid for x and y
+    x_space = linspace(x_lim(1), x_lim(2), size);
+    y_space = linspace(y_lim(1), y_lim(2), size);
+    [X, Y] = meshgrid(x_space, y_space);
+    
+    % Evaluate the function on the grid
+    for i = 1:size
+        for j = 1:size
+            % Pass each [x1; x2] as input to fun
+            Z(i, j) = fun([X(i, j); Y(i, j)]);
+        end
+    end
+    
+    % 3D plot
+    figure('Name', 'f(x,y)', 'NumberTitle', 'off');
+    set(gcf, 'Position', [100, 100, image_width, image_height]); % Set the figure size
+    surf(X, Y, Z);
+    
+    % Customize the plot
+    xlabel('x1'); % Label for x-axis
+    ylabel('x2'); % Label for y-axis
+    zlabel('f(x, y)'); % Label for z-axis
+    title(plot_title, 'Interpreter', 'latex', 'FontSize', 16); % Title of the plot
+    colorbar;
+
+    % save the figure
+    if strcmp(filename, '') == 0
+        print(gcf, filename, '-dpng', '-r300');
+    end
+end

Work 3/scripts/plotContour.m

@@ -0,0 +1,41 @@
+function plotContour(fun, x_lim, y_lim, size, plot_title, filename)
+    % plot the contour of a function
+    % fun:          The function to plot
+    % x_lim:        The range for x axis. ex: [-2, 2]
+    % y_lim:        The range for y axis. ex: [0, 2]
+    % size:         The number of points for each axis
+    % plot_title:   The latex title for the plot
+    %
+
+    global image_width, 
+global image_height;
+
+    % Generate a grid for x and y
+    x_space = linspace(x_lim(1), x_lim(2), size);
+    y_space = linspace(y_lim(1), y_lim(2), size);
+    [X, Y] = meshgrid(x_space, y_space);
+    
+    % Evaluate the function on the grid
+    for i = 1:size
+        for j = 1:size
+            % Pass each [x1; x2] as input to fun
+            Z(i, j) = fun([X(i, j); Y(i, j)]);
+        end
+    end
+    
+    % Contour
+    figure('Name', 'Contours of f(x1,x2)', 'NumberTitle', 'off');
+    set(gcf, 'Position', [100, 100, image_width, image_height]); % Set the figure size
+    contour(X, Y, Z);
+    
+    % Customize the plot
+    xlabel('x1'); % Label for x-axis
+    ylabel('x2'); % Label for y-axis
+    title(plot_title, 'Interpreter', 'latex', 'FontSize', 16); % Title of the plot
+    colorbar;
+
+    % save the figure
+    if strcmp(filename, '') == 0
+        print(gcf, filename, '-dpng', '-r300');
+    end
+end

Work 3/scripts/plotConvCompare.m

@@ -0,0 +1,54 @@
+function plotConvCompare(points_1, title_1, points_2, title_2, points_3, title_3, Min_point, plot_title, filename)
+    % 3D plots a function
+    % points:       The points to plot
+    % contur_fun:   The function for contour plot
+    % x_lim:        The range for x axis. ex: [-2, 2]
+    % y_lim:        The range for y axis. ex: [0, 2]
+    % size:         The number of points for each axis
+    % plot_title:   The latex title for the plot
+    % filename:     The filename to save the plot (if exists)
+    %
+    
+    global image_width, 
+    global image_height;
+
+    distances_1 = sqrt((points_1(1, :) - Min_point(1)).^2 + (points_1(2, :) - Min_point(2)).^2);
+    distances_2 = sqrt((points_2(1, :) - Min_point(1)).^2 + (points_2(2, :) - Min_point(2)).^2);
+    distances_3 = sqrt((points_3(1, :) - Min_point(1)).^2 + (points_3(2, :) - Min_point(2)).^2);
+
+    
+    % 2D plot
+    figure('Name', 'Convergence compare', 'NumberTitle', 'off');
+    set(gcf, 'Position', [100, 100, image_width, image_height]); % Set the figure size
+    title(plot_title, 'Interpreter', 'latex', 'FontSize', 16); % Title of the plot
+    
+    % One
+    subplot(3, 1, 1);
+    plot(distances_1, '-o');
+    % Customize the plot
+    ylabel(title_1, 'Interpreter', 'none');
+    xlabel('Step');
+    grid on
+
+    % One
+    subplot(3, 1, 2);
+    plot(distances_2, '-o');
+    % Customize the plot
+    ylabel(title_2, 'Interpreter', 'none');
+    xlabel('Step');
+    grid on
+
+    % One
+    subplot(3, 1, 3);
+    plot(distances_3, '-o');
+    % Customize the plot
+    ylabel(title_3, 'Interpreter', 'none');
+    xlabel('Step');
+    grid on
+
+
+    % save the figure
+    if strcmp(filename, '') == 0
+        print(gcf, filename, '-dpng', '-r300');
+    end
+end

Work 3/scripts/plotItersOverGamma.m

@@ -0,0 +1,28 @@
+function plotItersOverGamma(gamma, iterations, plot_title, filename)
+    % 3D plots a function
+    % fun:          The points to plot
+    % contur_fun:   The function for contour plot
+    % x_lim:        The range for x axis. ex: [-2, 2]
+    % y_lim:        The range for y axis. ex: [0, 2]
+    % size:         The number of points for each axis
+    % plot_title:   The latex title for the plot
+    % filename:     The filename to save the plot (if exists)
+    %
+    
+    global image_width, 
+    global image_height;
+
+    figure('Name', 'Iterations_over_gamma', 'NumberTitle', 'off');
+    set(gcf, 'Position', [100, 100, image_width, image_height]); % Set the figure size
+    plot(gamma, iterations, '*r', 'LineWidth', 2);
+
+    % Customize the plot
+    title(plot_title, 'Interpreter', 'latex', 'FontSize', 16); % Title of the plot
+    xlabel('\gamma') ;
+    ylabel('Iterations');
+
+    % save the figure
+    if strcmp(filename, '') == 0
+        print(gcf, filename, '-dpng', '-r300');
+    end
+end

Work 3/scripts/plotPointsOverContour.m

@@ -0,0 +1,48 @@
+function plotPointsOverContour(points, contour_fun, x_lim, y_lim, size, plot_title, filename)
+    % 3D plots a function
+    % points:       The points to plot
+    % contur_fun:   The function for contour plot
+    % x_lim:        The range for x axis. ex: [-2, 2]
+    % y_lim:        The range for y axis. ex: [0, 2]
+    % size:         The number of points for each axis
+    % plot_title:   The latex title for the plot
+    % filename:     The filename to save the plot (if exists)
+    %
+    
+    global image_width, 
+    global image_height;
+
+    % Generate a grid for x and y
+    x_space = linspace(x_lim(1), x_lim(2), size);
+    y_space = linspace(y_lim(1), y_lim(2), size);
+    [X, Y] = meshgrid(x_space, y_space);
+    
+    % Evaluate the function on the grid
+    for i = 1:size
+        for j = 1:size
+            % Pass each [x1; x2] as input to fun
+            Z(i, j) = contour_fun([X(i, j); Y(i, j)]);
+        end
+    end
+    
+    % 2D plot
+    figure('Name', '(x1,x2) convergence', 'NumberTitle', 'off');
+    set(gcf, 'Position', [100, 100, image_width, image_height]); % Set the figure size
+    plot(points(1, :), points(2, :), '-or');
+    hold on
+    contour(X, Y, Z);
+    % Customize the plot
+    xlim(x_lim);
+    ylim(y_lim);
+    xlabel('x1'); % Label for x-axis
+    ylabel('x2'); % Label for y-axis
+    grid on
+
+    title(plot_title, 'Interpreter', 'latex', 'FontSize', 16); % Title of the plot
+    colorbar;
+
+    % save the figure
+    if strcmp(filename, '') == 0
+        print(gcf, filename, '-dpng', '-r300');
+    end
+end